history of mathematics - National STEM Centre

i———
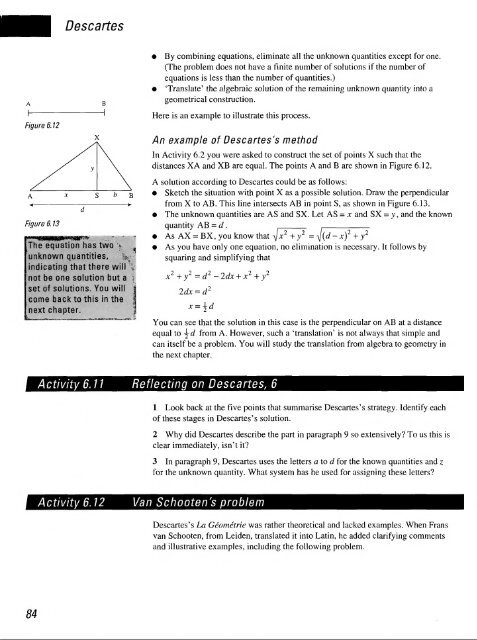
Figure 6.12
Figure 6.13
Descartes
re equanon has two
unknown quantities,
indicating that there will
not be one solution but a
set of solutions. You will
come back to this in the
next chapter.
84
• By combining equations, eliminate all the unknown quantities except for one.
(The problem does not have a finite number of solutions if the number of
equations is less than the number of quantities.)
• 'Translate' the algebraic solution of the remaining unknown quantity into a
geometrical construction.
Here is an example to illustrate this process.
An example of Descartes's method
In Activity 6.2 you were asked to construct the set of points X such that the
distances XA and XB are equal. The points A and B are shown in Figure 6.12.
A solution according to Descartes could be as follows:
• Sketch the situation with point X as a possible solution. Draw the perpendicular
from X to AB. This line intersects AB in point S, as shown in Figure 6.13.
• The unknown quantities are AS and SX. Let AS = x and SX = y, and the known
quantity AB = d . ____ _______
• As AX = BX, you know that -\x 2 +y 2 = -y(d - x) 2 + y 2
• As you have only one equation, no elimination is necessary. It follows by
squaring and simplifying that
2 2 i2 --» 2 2
x + y = d - 2dx + x + y
2dx = d 2
You can see that the solution in this case is the perpendicular on AB at a distance
equal to j d from A. However, such a 'translation' is not always that simple and
can itself be a problem. You will study the translation from algebra to geometry in
the next chapter.
Activity 6.11 Reflecting on Descartes, 6
Activity 6.12 Van Schooten's problem
1 Look back at the five points that summarise Descartes's strategy. Identify each
of these stages in Descartes's solution.
2 Why did Descartes describe the part in paragraph 9 so extensively? To us this is
clear immediately, isn't it?
3 In paragraph 9, Descartes uses the letters a to d for the known quantities and z
for the unknown quantity. What system has he used for assigning these letters?
Descartes's La Geometric was rather theoretical and lacked examples. When Frans
van Schooten, from Leiden, translated it into Latin, he added clarifying comments
and illustrative examples, including the following problem.