On the Flavor Problem in Strongly Coupled Theories - THEP Mainz

76 Chapter 2. The Randall Sundrum Model and its Holographic Interpretation
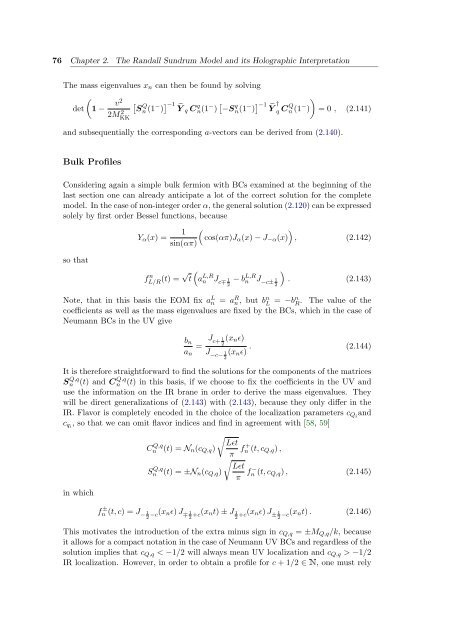
The mass eigenvalues xn can then be found by solving
�
det 1 − v2
2M 2 � Q
Sn (1
KK
− ) �−1 �Y
q C q n(1 − ) � −S q n(1 − ) �−1 †
�Y
q C Q n (1 − �
) = 0 , (2.141)
and subsequentially the corresponding a-vectors can be derived from (2.140).
Bulk Profiles
Considering again a simple bulk fermion with BCs examined at the beginning of the
last section one can already anticipate a lot of the correct solution for the complete
model. In the case of non-integer order α, the general solution (2.120) can be expressed
solely by first order Bessel functions, because
so that
Yα(x) =
1
�
�
cos(απ)Jα(x) − J−α(x) , (2.142)
sin(απ)
f n L/R (t) = √ �
t a L,R
n J 1
c∓ − b
2
L,R
�
n J 1
−c± . (2.143)
2
Note, that in this basis the EOM fix aL n = aR n , but bn L = −bn R . The value of the
coefficients as well as the mass eigenvalues are fixed by the BCs, which in the case of
Neumann BCs in the UV give
bn
an
= Jc+ 1 (xnɛ)
2
J 1
−c− (xnɛ)
2
. (2.144)
It is therefore straightforward to find the solutions for the components of the matrices
SQ,q n (t) and CQ,q n (t) in this basis, if we choose to fix the coefficients in the UV and
use the information on the IR brane in order to derive the mass eigenvalues. They
will be direct generalizations of (2.143) with (2.143), because they only differ in the
IR. Flavor is completely encoded in the choice of the localization parameters cQiand , so that we can omit flavor indices and find in agreement with [58, 59]
cqi
in which
C Q,q
�
Lɛt
n (t) = Nn(cQ,q)
S Q,q
n (t) = ±Nn(cQ,q)
π f + n (t, cQ,q) ,
� Lɛt
π f − n (t, cQ,q) , (2.145)
f ± n (t, c) = J − 1
2 −c(xnɛ) J ∓ 1
2 +c(xnt) ± J 1
2 +c(xnɛ) J ± 1
2 −c(xnt) . (2.146)
This motivates the introduction of the extra minus sign in cQ,q = ±MQ,q/k, because
it allows for a compact notation in the case of Neumann UV BCs and regardless of the
solution implies that cQ,q < −1/2 will always mean UV localization and cQ,q > −1/2
IR localization. However, in order to obtain a profile for c + 1/2 ∈ �, one must rely