The mechanical effects of short-circuit currents in - Montefiore

(2.26)
M
el-pl
⎡ y
d/
2
y
= 2⎢
σ yb y + σ
⎢∫
F d
y ∫
⎣0
y
= σ
F
⎡
d
⎢
⎛ ⎞
⎜ ⎟
⎢
⎣
⎝ 2 ⎠
2
2
y
⎤
− ⎥ b
3 ⎥
⎦
F
⎤
yb d y⎥
⎥
⎦
When y = 0 the flow stress is in the complete cross
section, Figure 2.1d. With a permanent lengthening in
the outer fibre of ε0,2 = 0,2 %, an outer moment MTr acts
which is called realistic ultimate load. It is equal to the
inner moment Mel-pl according to equation (2.26):
(2.27)
M
Tr
= M
el-pl
= 1,
5Z
m
( y = 0)
σ
0,2
m
2
d b
= σ
4
= qZ σ
In contrast to the pure elastic limit the outer moment can
be increased about 50 %. Then the load capacity is
exhausted. The perfect plastic factor is q = 1,5 for
rectangular profiles. With other profiles factor q
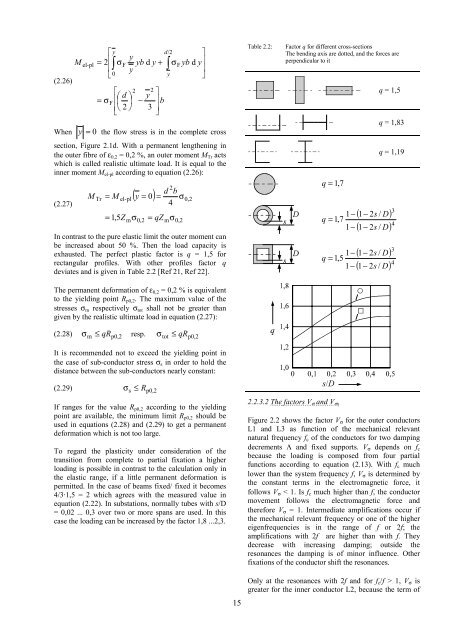
deviates and is given in Table 2.2 [Ref 21, Ref 22].
The permanent deformation of ε0,2 = 0,2 % is equivalent
to the yielding point Rp0,2. The maximum value of the
stresses σm respectively σtot shall not be greater than
given by the realistic ultimate load in equation (2.27):
0,2
0,2
(2.28) σ m ≤ qRp0,2
resp. σ tot ≤ qRp0,2
It is recommended not to exceed the yielding point in
the case of sub-conductor stress σs in order to hold the
distance between the sub-conductors nearly constant:
R ≤
(2.29) s p0,2
σ
If ranges for the value Rp0,2 according to the yielding
point are available, the minimum limit Rp0,2 should be
used in equations (2.28) and (2.29) to get a permanent
deformation which is not too large.
To regard the plasticity under consideration of the
transition from complete to partial fixation a higher
loading is possible in contrast to the calculation only in
the elastic range, if a little permanent deformation is
permitted. In the case of beams fixed/ fixed it becomes
4/3·1,5 = 2 which agrees with the measured value in
equation (2.22). In substations, normally tubes with s/D
= 0,02 ... 0,3 over two or more spans are used. In this
case the loading can be increased by the factor 1,8 ...2,3.
15
Table 2.2: Factor q for different cross-sections
The bending axis are dotted, and the forces are
perpendicular to it
q = 1,
7
1−
q = 1,
7
1−
1−
q = 1,
5
1−
2.2.3.2 The factors V
σ and V
σs
q = 1,5
q = 1,83
q = 1,19
( )
( ) 4
3
1−
2s
/ D
1−
2s
/ D
( )
( ) 4
3
1−
2s
/ D
1−
2s
/ D
Figure 2.2 shows the factor Vσ for the outer conductors
L1 and L3 as function of the mechanical relevant
natural frequency fc of the conductors for two damping
decrements Λ and fixed supports. Vσ depends on fc
because the loading is composed from four partial
functions according to equation (2.13). With fc much
lower than the system frequency f, Vσ is determined by
the constant terms in the electromagnetic force, it
follows Vσ < 1. Is fc much higher than f, the conductor
movement follows the electromagnetic force and
therefore Vσ = 1. Intermediate amplifications occur if
the mechanical relevant frequency or one of the higher
eigenfrequencies is in the range of f or 2f; the
amplifications with 2f are higher than with f. They
decrease with increasing damping; outside the
resonances the damping is of minor influence. Other
fixations of the conductor shift the resonances.
Only at the resonances with 2f and for fc/f > 1, Vσ is
greater for the inner conductor L2, because the term of