Applied numerical modeling of saturated / unsaturated flow and ...
Applied numerical modeling of saturated / unsaturated flow and ...
Applied numerical modeling of saturated / unsaturated flow and ...
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
where vmax [s -1 ] is a maximum growth rate,<br />
Ci [kg m -3 ] is the concentration <strong>of</strong> the i th<br />
substrate, MCi [kg m -3 ] is the corresponding<br />
half velocity concentration [kg m -3 ], Cj<br />
[kg m -3 ] is the concentration <strong>of</strong> the j th substance<br />
inhibiting microbial growth, ICj is the<br />
corresponding inhibition concentration<br />
[kg m -3 ] <strong>and</strong> �(X) is a microbial decay term,<br />
which is <strong>of</strong>ten modeled as being <strong>of</strong> first<br />
order. Consumption <strong>of</strong> substrates or production<br />
<strong>of</strong> k metabolites Ck [kg m -3 ] is both<br />
coupled to microbial growth via (Schäfer et<br />
al., 1998)<br />
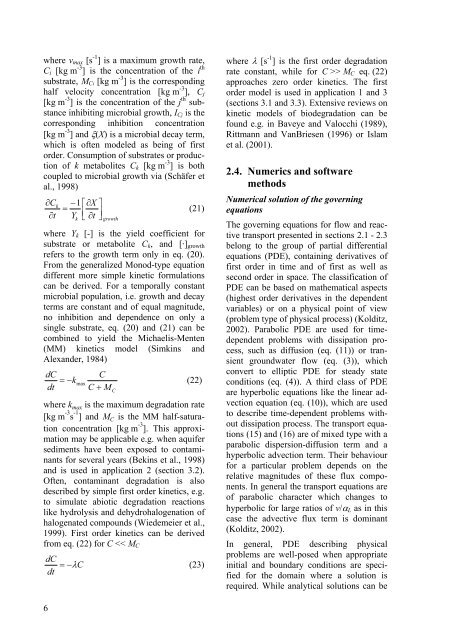
�Ck �1<br />
��X<br />
�<br />
�<br />
�t<br />
Y �<br />
� �t<br />
�<br />
�<br />
6<br />
(21)<br />
k growth<br />
where Yk [-] is the yield coefficient for<br />
substrate or metabolite Ck, <strong>and</strong> [·]growth<br />
refers to the growth term only in eq. (20).<br />
From the generalized Monod-type equation<br />
different more simple kinetic formulations<br />
can be derived. For a temporally constant<br />
microbial population, i.e. growth <strong>and</strong> decay<br />
terms are constant <strong>and</strong> <strong>of</strong> equal magnitude,<br />
no inhibition <strong>and</strong> dependence on only a<br />
single substrate, eq. (20) <strong>and</strong> (21) can be<br />
combined to yield the Michaelis-Menten<br />
(MM) kinetics model (Simkins <strong>and</strong><br />
Alex<strong>and</strong>er, 1984)<br />
dC<br />
dt<br />
C<br />
� �kmax<br />
(22)<br />
C � M C<br />
where k max is the maximum degradation rate<br />
[kg m -3 s -1 ] <strong>and</strong> M C is the MM half-saturation<br />
concentration [kg m -3 ]. This approximation<br />
may be applicable e.g. when aquifer<br />
sediments have been exposed to contaminants<br />
for several years (Bekins et al., 1998)<br />
<strong>and</strong> is used in application 2 (section 3.2).<br />
Often, contaminant degradation is also<br />
described by simple first order kinetics, e.g.<br />
to simulate abiotic degradation reactions<br />
like hydrolysis <strong>and</strong> dehydrohalogenation <strong>of</strong><br />
halogenated compounds (Wiedemeier et al.,<br />
1999). First order kinetics can be derived<br />
from eq. (22) for C > MC eq. (22)<br />
approaches zero order kinetics. The first<br />
order model is used in application 1 <strong>and</strong> 3<br />
(sections 3.1 <strong>and</strong> 3.3). Extensive reviews on<br />
kinetic models <strong>of</strong> biodegradation can be<br />
found e.g. in Baveye <strong>and</strong> Valocchi (1989),<br />
Rittmann <strong>and</strong> VanBriesen (1996) or Islam<br />
et al. (2001).<br />
2.4. Numerics <strong>and</strong> s<strong>of</strong>tware<br />
methods<br />
Numerical solution <strong>of</strong> the governing<br />
equations<br />
The governing equations for <strong>flow</strong> <strong>and</strong> reactive<br />
transport presented in sections 2.1 - 2.3<br />
belong to the group <strong>of</strong> partial differential<br />
equations (PDE), containing derivatives <strong>of</strong><br />
first order in time <strong>and</strong> <strong>of</strong> first as well as<br />
second order in space. The classification <strong>of</strong><br />
PDE can be based on mathematical aspects<br />
(highest order derivatives in the dependent<br />
variables) or on a physical point <strong>of</strong> view<br />
(problem type <strong>of</strong> physical process) (Kolditz,<br />
2002). Parabolic PDE are used for timedependent<br />
problems with dissipation process,<br />
such as diffusion (eq. (11)) or transient<br />
groundwater <strong>flow</strong> (eq. (3)), which<br />
convert to elliptic PDE for steady state<br />
conditions (eq. (4)). A third class <strong>of</strong> PDE<br />
are hyperbolic equations like the linear advection<br />
equation (eq. (10)), which are used<br />
to describe time-dependent problems without<br />
dissipation process. The transport equations<br />
(15) <strong>and</strong> (16) are <strong>of</strong> mixed type with a<br />
parabolic dispersion-diffusion term <strong>and</strong> a<br />
hyperbolic advection term. Their behaviour<br />
for a particular problem depends on the<br />
relative magnitudes <strong>of</strong> these flux components.<br />
In general the transport equations are<br />
<strong>of</strong> parabolic character which changes to<br />
hyperbolic for large ratios <strong>of</strong> v/�L as in this<br />
case the advective flux term is dominant<br />
(Kolditz, 2002).<br />
In general, PDE describing physical<br />
problems are well-posed when appropriate<br />
initial <strong>and</strong> boundary conditions are specified<br />
for the domain where a solution is<br />
required. While analytical solutions can be