1 Montgomery Modular Multiplication in Hard- ware
1 Montgomery Modular Multiplication in Hard- ware
1 Montgomery Modular Multiplication in Hard- ware
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
FEI KEMT<br />
the ECC <strong>in</strong>stead of the RSA or DSA [56] lies <strong>in</strong> the fact that the length of key<br />
can be much shorter. The best known algorithm for solv<strong>in</strong>g the elliptic curve dis-<br />
crete logarithm problem (ECDLP) takes fully exponential time, while the algorithms<br />
for the <strong>in</strong>teger factorization problem and the discrete logarithm problem take sub-<br />
exponential time. The comparison of key length for equivalent security level is<br />
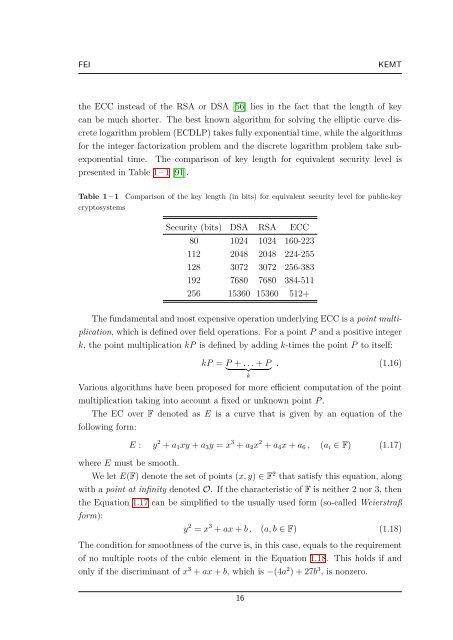
presented <strong>in</strong> Table 1 – 1 [91].<br />
Table 1 – 1 Comparison of the key length (<strong>in</strong> bits) for equivalent security level for public-key<br />
cryptosystems<br />
Security (bits) DSA RSA ECC<br />
80 1024 1024 160-223<br />
112 2048 2048 224-255<br />
128 3072 3072 256-383<br />
192 7680 7680 384-511<br />
256 15360 15360 512+<br />
The fundamental and most expensive operation underly<strong>in</strong>g ECC is a po<strong>in</strong>t multi-<br />
plication, which is def<strong>in</strong>ed over field operations. For a po<strong>in</strong>t P and a positive <strong>in</strong>teger<br />
k, the po<strong>in</strong>t multiplication kP is def<strong>in</strong>ed by add<strong>in</strong>g k-times the po<strong>in</strong>t P to itself:<br />
kP = P + . . . + P<br />
� �� �<br />
k<br />
. (1.16)<br />
Various algorithms have been proposed for more efficient computation of the po<strong>in</strong>t<br />
multiplication tak<strong>in</strong>g <strong>in</strong>to account a fixed or unknown po<strong>in</strong>t P .<br />
The EC over F denoted as E is a curve that is given by an equation of the<br />
follow<strong>in</strong>g form:<br />
where E must be smooth.<br />
E : y 2 + a1xy + a3y = x 3 + a2x 2 + a4x + a6 , (ai ∈ F) (1.17)<br />
We let E(F) denote the set of po<strong>in</strong>ts (x, y) ∈ F 2 that satisfy this equation, along<br />
with a po<strong>in</strong>t at <strong>in</strong>f<strong>in</strong>ity denoted O. If the characteristic of F is neither 2 nor 3, then<br />
the Equation 1.17 can be simplified to the usually used form (so-called Weierstraß<br />
form):<br />
y 2 = x 3 + ax + b . (a, b ∈ F) (1.18)<br />
The condition for smoothness of the curve is, <strong>in</strong> this case, equals to the requirement<br />
of no multiple roots of the cubic element <strong>in</strong> the Equation 1.18. This holds if and<br />
only if the discrim<strong>in</strong>ant of x 3 + ax + b, which is −(4a 2 ) + 27b 3 , is nonzero.<br />
16