Derivada de una función - TEC-Digital

60 Capítulo 2: Derivadas
Ejemplo 5
(x − 3)2
Por último verifiquemos que +
9
y2
= 1 es una ecuación de la relación determinada por las ecuaciones
4
paramétricas x = 3(1 − cos θ) , y = 2 sen θ, con θ ∈ R.
Como x = 3(1 − cos θ) entonces cos θ = 1 − x
y
, y como y = 2 sen θ entonces sen θ =
3 2
Luego sen2 θ + cos2
y
2 θ = + (1 −
2
x
3 )2 , de donde 1 = y2 (x − 3)2
+ , que es la ecuación de una elipse con
4 9
centro en (3, 0).
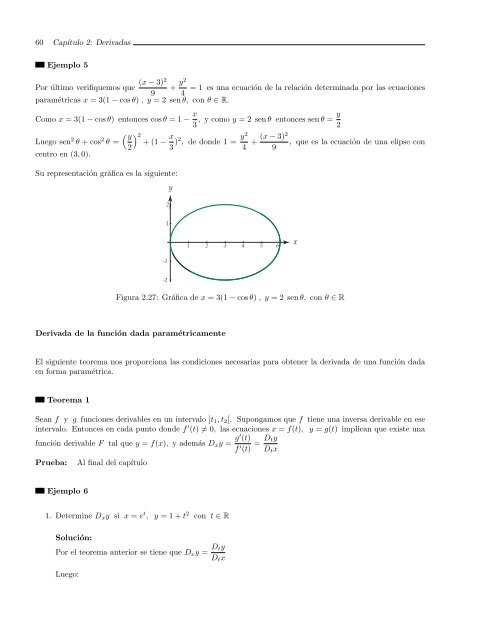
Su representación gráfica es la siguiente:
Figura 2.27: Gráfica de x = 3(1 − cos θ) , y = 2 sen θ, con θ ∈ R
Derivada de la función dada paramétricamente
El siguiente teorema nos proporciona las condiciones necesarias para obtener la derivada de una función dada
en forma paramétrica.
Teorema 1
Sean f y g funciones derivables en un intervalo ]t1, t2[. Supongamos que f tiene una inversa derivable en ese
intervalo. Entonces en cada punto donde f ′ (t) = 0, las ecuaciones x = f(t), y = g(t) implican que existe una
función derivable F tal que y = f(x), y además Dxy = g′ (t)
f ′ Dty
=
(t) Dtx
Prueba: Al final del capítulo
Ejemplo 6
1. Determine Dxy si x = e t , y = 1 + t 2 con t ∈ R
Solución:
Por el teorema anterior se tiene que Dxy = Dty
Dtx
Luego: