10. Appendix

Solution to Problem 3.11(a,b and c)
Solution to Problem 3.11(a,b and c) 611
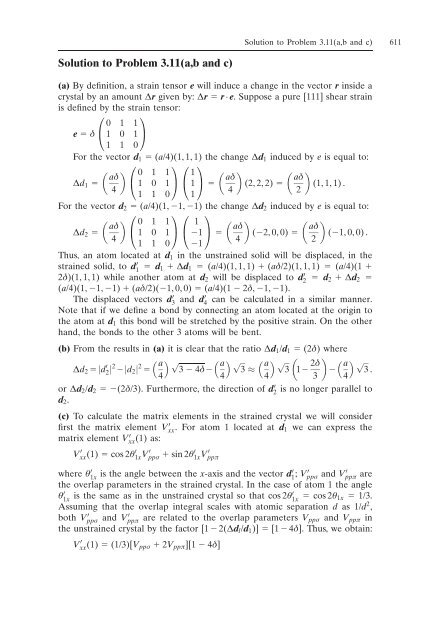
(a) By definition, a strain tensor e will induce a change in the vector r inside a
crystal by an amount ¢r given by: ¢r r · e. Suppose a pure [111] shear strain
is defined by the strain tensor:
⎛ ⎞
0 1 1
e ‰ ⎝ 1 0 1⎠
1 1 0
For the vector d1 (a/4)(1, 1, 1) the change ¢d1 induced by e is equal to:
a‰
¢d1
4
⎛ ⎞ ⎛
0 1 1
⎝ 1 0 1⎠⎝
1 1 0
1
⎞
1 ⎠
a‰
a‰
(2, 2, 2) (1, 1, 1) .
4
2
1
For the vector d2 (a/4)(1, 1, 1) the change ¢d2 induced by e is equal to:
a‰
¢d2
4
⎛ ⎞ ⎛
0 1 1
⎝ 1 0 1⎠⎝
1 1 0
1
⎞
1 ⎠
a‰
a‰
(2, 0, 0) (1, 0, 0) .
4
2
1
Thus, an atom located at d1 in the unstrained solid will be displaced, in the
strained solid, to d ′ 1 d1 ¢d1 (a/4)(1, 1, 1) (a‰/2)(1, 1, 1) (a/4)(1
2‰)(1, 1, 1) while another atom at d2 will be displaced to d ′ 2 d2 ¢d2
(a/4)(1, 1, 1) (a‰/2)(1, 0, 0) (a/4)(1 2‰, 1, 1).
The displaced vectors d ′ 3 and d′ 4 can be calculated in a similar manner.
Note that if we define a bond by connecting an atom located at the origin to
the atom at d1 this bond will be stretched by the positive strain. On the other
hand, the bonds to the other 3 atoms will be bent.
(b) From the results in (a) it is clear that the ratio ¢d1/d1 (2‰) where
¢d2 |d ′ 2| 2 |d2| 2
a
√
a
√
a
√
3 4‰ 3 ≈ 3 1
4
4 4
2‰
a
√
3.
3 4
or ¢d2/d2 (2‰/3). Furthermore, the direction of d ′ 2 is no longer parallel to
d2.
(c) To calculate the matrix elements in the strained crystal we will consider
first the matrix element V ′ xx . For atom 1 located at d1 we can express the
matrix element V ′ xx (1) as:
V ′ xx (1) cos 2ı′ 1x V′ ppÛ sin 2ı′ 1x V′ ppapple
where ı ′ 1x is the angle between the x-axis and the vector d′ 1 ; V′ ppÛ and V′ ppapple are
the overlap parameters in the strained crystal. In the case of atom 1 the angle
ı ′ 1x is the same as in the unstrained crystal so that cos 2ı′ 1x cos 2ı1x 1/3.
Assuming that the overlap integral scales with atomic separation d as 1/d 2 ,
both V ′ ppÛ and V′ ppapple are related to the overlap parameters VppÛ and Vppapple in
the unstrained crystal by the factor [1 2(¢dl/d1)] [1 4‰]. Thus, we obtain:
V ′ xx (1) (1/3)[VppÛ 2Vppapple][1 4‰]