Application and Optimisation of the Spatial Phase Shifting ...

4.2 Noise quantification in this work 107
A simplex in IR n is a (hyper-)body set up by n+1 vertices; it is the simplest body one can create in the
respective dimensionality. In IR 3 , a simplex is a tetrahedron. Because this is the parameter space that we
are in with our type of sawtooth images, we consider this example to clarify how the strategy works.
Initially, the routine is passed a starting vertex, which is the user guess for (N x , N y , N 0 ). From this, the
noise-free fringe system ∆ϕ ref(x,y) is calculated to compare it with ∆ϕ meas(x,y). The resulting σ ∆ϕ is
assigned to the first vertex. Then, the three other vertices are established by simply varying each one of
the parameter co-ordinates a little; this 3-bein ensures that a volume is generated instead of a plane or a
line. Each of the vertices defines a slightly different ∆ϕ ref(x,y) and thus leads to its corresponding σ ∆ϕ , so
that we have a set of four different σ ∆ϕ . The vertex that has generated σ ∆ϕ,max is the worst-fitting point,
and hence the one to move through the IR 3 to find a location closer to the minimum for it. (There are many
local minima, but with an accuracy of ¼ fringe for the starting values, the absolute minimum is safely
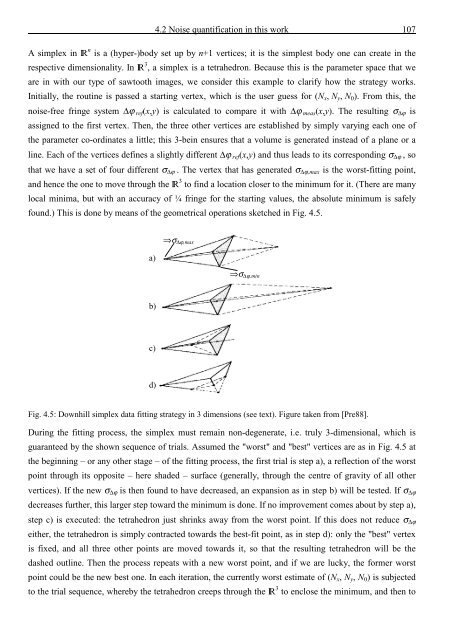
found.) This is done by means of the geometrical operations sketched in Fig. 4.5.
a)
⇒σ ∆ϕ,max
⇒σ ∆ϕ,min
b)
c)
d)
Fig. 4.5: Downhill simplex data fitting strategy in 3 dimensions (see text). Figure taken from [Pre88].
During the fitting process, the simplex must remain non-degenerate, i.e. truly 3-dimensional, which is
guaranteed by the shown sequence of trials. Assumed the "worst" and "best" vertices are as in Fig. 4.5 at
the beginning – or any other stage – of the fitting process, the first trial is step a), a reflection of the worst
point through its opposite – here shaded – surface (generally, through the centre of gravity of all other
vertices). If the new σ ∆ϕ is then found to have decreased, an expansion as in step b) will be tested. If σ ∆ϕ
decreases further, this larger step toward the minimum is done. If no improvement comes about by step a),
step c) is executed: the tetrahedron just shrinks away from the worst point. If this does not reduce σ ∆ϕ
either, the tetrahedron is simply contracted towards the best-fit point, as in step d): only the "best" vertex
is fixed, and all three other points are moved towards it, so that the resulting tetrahedron will be the
dashed outline. Then the process repeats with a new worst point, and if we are lucky, the former worst
point could be the new best one. In each iteration, the currently worst estimate of (N x , N y , N 0 ) is subjected
to the trial sequence, whereby the tetrahedron creeps through the IR 3 to enclose the minimum, and then to