Online proceedings - EDA Publishing Association

7-9 October 2009, Leuven, Belgium
parameterization of the shape is necessary. This is realized
by defining the shape on a number of equidistant locations
along the whole channel length. A piece-wise linear shape
profile is then assumed between these points. Thus,
intermediate values can be obtained by interpolation.
This method has the advantages that it resolves the profile
at a high spatial accuracy and that every variable has only
local influence on the shape. This is in contrast with the
parameterization that was used in [1], where the shape is
represented by a smooth quadratic polynomial function. The
coefficients of this polynomial were used as variables subject
to optimization. Every variable has therefore an influence
along the whole channel length. As we will show later on, the
thermal resistance minimization shows a non-smooth optimal
shape profile that cannot be captured by the parameterization
by [1].
The optimization problems (7) and (8) are solved
numerically using a conjugate gradient method.
IV.
RESULTS AND DISCUSSION
A. Minimal wall temperature gradient – minimization of J
1
Optimization of the microchannel heat sink is performed on
an illustrative case, using a channel height of 600 µm,
minimal fin width of 30 µm and chip length of 1 cm. The
pressure drop is fixed at 0.6 bar and water was used as a
coolant. Material properties are evaluated at 20 °C. This
gives rise to the following model parameters: w = 0. 05 and
f
−6
χ = 1.851⋅10
. The simplified model without axial
conduction (6) is used since in the optimum there is nearly no
axial temperature gradient and thus negligible axial
conduction. In addition this reduces the required
computational effort.
When optimizing with respect to a minimal temperature
gradient, using objective function J 1
, it is observed that a
unique solution does not exist in particular circumstances.
This occurs when the resulting shape has uniform wall
temperature, which is the absolute optimum. We will refer to
this later.
A similar observation was encountered in [1]. To
circumvent the non-uniqueness of the optimization problem,
Bau made a linear combination with the thermal resistance
objective function using a weighting factor. This
methodology resulted in a well-posed optimization problem,
but no justification on the choice of the weighting factor was
made.
In order to keep the distinction between the two objectives,
we perform the optimization in another way. In our research,
the inlet width and its corresponding aspect ratio α are set to
0
a fixed value. Since this value is directly related to the
resulting wall temperature, the optimal solution becomes
unique.
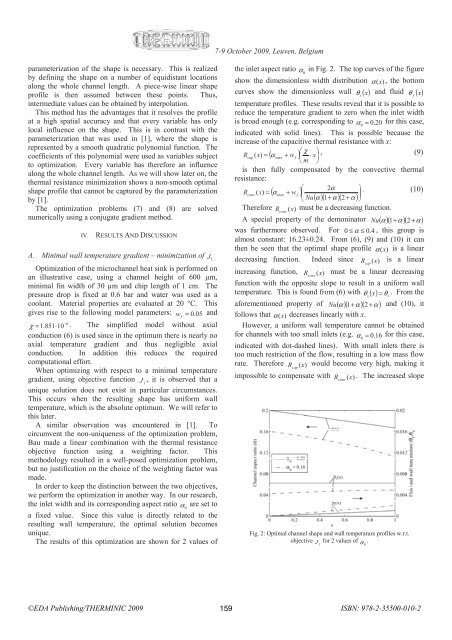
The results of this optimization are shown for 2 values of
the inlet aspect ratio α in Fig. 2. The top curves of the figure
0
show the dimensionless width distribution α (x), the bottom
curves show the dimensionless wall θ
s
( x)
and fluid θ
f
( x)
temperature profiles. These results reveal that it is possible to
reduce the temperature gradient to zero when the inlet width
is broad enough (e.g. corresponding to α = 0. 0
20 for this case,
indicated with solid lines). This is possible because the
increase of the capacitive thermal resistance with x:
⎛ χ ⎞
Rcap
( x)
= ( α + w
f
) ⎜ ⋅ x⎟ , (9)
max
⎝ m ⎠
is then fully compensated by the convective thermal
resistance:
⎛ 2α
( )
( )( )( ) ⎟ ⎞
R ( x)
= α
max
+ w ⎜
. (10)
conv
f
⎝ Nu α 1+
α 2 + α ⎠
Therefore R conv
(x)
must be a decreasing function.
A special property of the demoninator Nu ( α )( 1+ α )( 2 + α )
was furthermore observed. For 0 ≤ α ≤ 0. 4 , this group is
almost constant: 16.23±0.24. From (6), (9) and (10) it can
then be seen that the optimal shape profile α (x)
is a linear
decreasing function. Indeed since R cap
(x)
is a linear
increasing function, R conv
(x)
must be a linear decreasing
function with the opposite slope to result in a uniform wall
temperature. This is found from (6) with θ
s( x) = θ . From the
s
aforementioned property of Nu ( α )( 1+ α )( 2 + α ) and (10), it
follows that α (x)
decreases linearly with x.
However, a uniform wall temperature cannot be obtained
for channels with too small inlets (e.g. α = 0. 0
16 for this case,
indicated with dot-dashed lines). With small inlets there is
too much restriction of the flow, resulting in a low mass flow
rate. Therefore R cap
(x)
would become very high, making it
impossible to compensate with R conv
(x)
. The increased slope
Fig. 2: Optimal channel shape and wall temperature profiles w.r.t.
objective J for 2 values of
1
α .
0
©EDA Publishing/THERMINIC 2009 159
ISBN: 978-2-35500-010-2