Abrir - RDU - Universidad Nacional de Córdoba

APÉNDICE 91
A n :
✟◦ ❍ + ❍❍❍❍
◦ ✟✟✟✟✟ ◦ ◦ . . . ◦ ❍◦ B n :
◦ +
✏
◦
✏✏ ◦ . . . ◦ ◦ ◦
C n : ◦
+
◦ . . . ◦ ◦ ◦
E 6 :
◦ +
◦
✏ ◦ ✏✏
✏◦
◦
✏✏
D n :
◦ ◦ E 7 : ◦ ◦ ◦
◦ +
✏
◦
✏✏ ◦ . . . ◦ ◦ ✏✏ ✏◦
◦
◦
◦
◦ ◦ ◦ ◦ +
E 8 : ◦
+
◦ ◦ ◦ ◦ ◦
◦ ◦
F 4 : ◦ ◦ ◦ ◦ ◦
+
G 2 : ◦ ◦ ◦
+
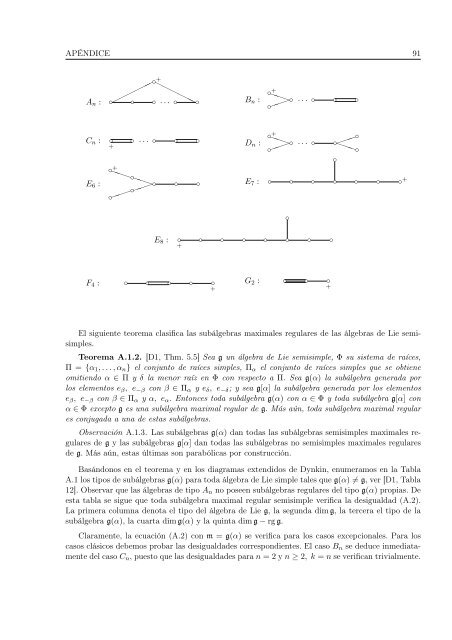
El siguiente teorema clasifica las subálgebras maximales regulares de las álgebras de Lie semisimples.
Teorema A.1.2. [D1, Thm. 5.5] Sea g un álgebra de Lie semisimple, Φ su sistema de raíces,
Π = {α 1 , . . . , α n } el conjunto de raíces simples, Π α el conjunto de raíces simples que se obtiene
omitiendo α ∈ Π y δ la menor raíz en Φ con respecto a Π. Sea g(α) la subálgebra generada por
los elementos e β , e −β con β ∈ Π α y e δ , e −δ ; y sea g[α] la subálgebra generada por los elementos
e β , e −β con β ∈ Π α y α, e α . Entonces toda subálgebra g(α) con α ∈ Φ y toda subálgebra g[α] con
α ∈ Φ excepto g es una subálgebra maximal regular de g. Más aún, toda subálgebra maximal regular
es conjugada a una de estas subálgebras.
Observación A.1.3. Las subálgebras g(α) dan todas las subálgebras semisimples maximales regulares
de g y las subálgebras g[α] dan todas las subálgebras no semisimples maximales regulares
de g. Más aún, estas últimas son parabólicas por construcción.
Basándonos en el teorema y en los diagramas extendidos de Dynkin, enumeramos en la Tabla
A.1 los tipos de subálgebras g(α) para toda álgebra de Lie simple tales que g(α) ≠ g, ver [D1, Tabla
12]. Observar que las álgebras de tipo A n no poseen subálgebras regulares del tipo g(α) propias. De
esta tabla se sigue que toda subálgebra maximal regular semisimple verifica la desigualdad (A.2).
La primera columna denota el tipo del álgebra de Lie g, la segunda dim g, la tercera el tipo de la
subálgebra g(α), la cuarta dim g(α) y la quinta dim g − rg g.
Claramente, la ecuación (A.2) con m = g(α) se verifica para los casos excepcionales. Para los
casos clásicos debemos probar las desigualdades correspondientes. El caso B n se deduce inmediatamente
del caso C n , puesto que las desigualdades para n = 2 y n ≥ 2, k = n se verifican trivialmente.