Aspect in Ancient Greek - Nijmegen Centre for Semantics

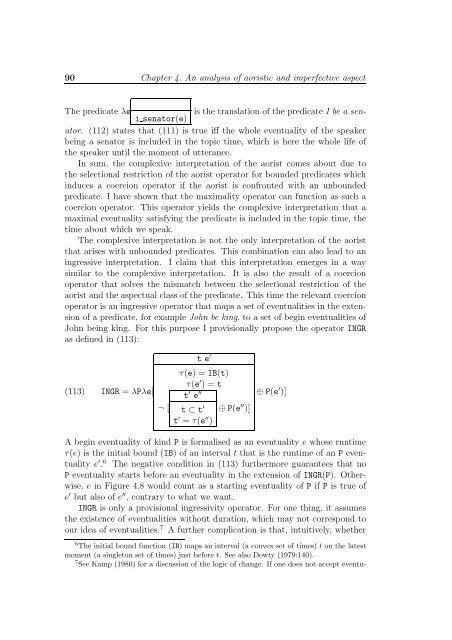
90 Chapter 4. An analysis of aoristic and imperfective aspectThe predicate λe i senator(e)is the translation of the predicate I be a senator.(112) states that (111) is true iff the whole eventuality of the speakerbeing a senator is included in the topic time, which is here the whole life ofthe speaker until the moment of utterance.In sum, the complexive interpretation of the aorist comes about due tothe selectional restriction of the aorist operator for bounded predicates whichinduces a coercion operator if the aorist is confronted with an unboundedpredicate. I have shown that the maximality operator can function as such acoercion operator. This operator yields the complexive interpretation that amaximal eventuality satisfying the predicate is included in the topic time, thetime about which we speak.The complexive interpretation is not the only interpretation of the aoristthat arises with unbounded predicates. This combination can also lead to aningressive interpretation. I claim that this interpretation emerges in a waysimilar to the complexive interpretation. It is also the result of a coercionoperator that solves the mismatch between the selectional restriction of theaorist and the aspectual class of the predicate. This time the relevant coercionoperator is an ingressive operator that maps a set of eventualities in the extensionof a predicate, for example John be king, to a set of begin eventualities ofJohn being king. For this purpose I provisionally propose the operator INGRas defined in (113):(113) INGR = λPλe[¬ [t e ′τ(e) = IB(t)τ(e ′ ) = tt ′ e ′′t ⊂ t ′t ′ = τ(e ′′ )⊕P(e ′′ )]⊕P(e ′ )]A begin eventuality of kind P is formalised as an eventuality e whose runtimeτ(e) is the initial bound (IB) of an interval t that is the runtime of an P eventualitye ′ . 6 The negative condition in (113) furthermore guarantees that noP eventuality starts before an eventuality in the extension of INGR(P). Otherwise,e in Figure 4.8 would count as a starting eventuality of P if P is true ofe ′ but also of e ′′ , contrary to what we want.INGR is only a provisional ingressivity operator. For one thing, it assumesthe existence of eventualities without duration, which may not correspond toour idea of eventualities. 7 A further complication is that, intuitively, whether6 The initial bound function (IB) maps an interval (a convex set of times) t on the latestmoment (a singleton set of times) just before t. See also Dowty (1979:140).7 See Kamp (1980) for a discussion of the logic of change. If one does not accept eventu-