Aspect in Ancient Greek - Nijmegen Centre for Semantics

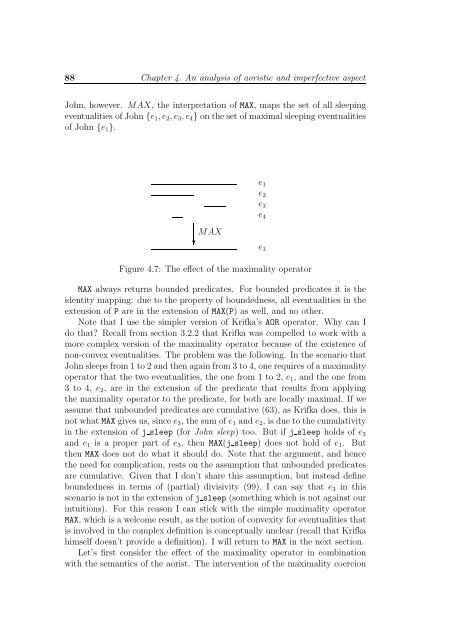
88 Chapter 4. An analysis of aoristic and imperfective aspectJohn, however. MAX, the interpretation of MAX, maps the set of all sleepingeventualities of John {e 1 , e 2 , e 3 , e 4 } on the set of maximal sleeping eventualitiesof John {e 1 }.e 1e 2e 3e 4MAX❄e 1Figure 4.7: The effect of the maximality operatorMAX always returns bounded predicates. For bounded predicates it is theidentity mapping: due to the property of boundedness, all eventualities in theextension of P are in the extension of MAX(P) as well, and no other.Note that I use the simpler version of Krifka’s AOR operator. Why can Ido that? Recall from section 3.2.2 that Krifka was compelled to work with amore complex version of the maximality operator because of the existence ofnon-convex eventualities. The problem was the following. In the scenario thatJohn sleeps from 1 to 2 and then again from 3 to 4, one requires of a maximalityoperator that the two eventualities, the one from 1 to 2, e 1 , and the one from3 to 4, e 2 , are in the extension of the predicate that results from applyingthe maximality operator to the predicate, for both are locally maximal. If weassume that unbounded predicates are cumulative (63), as Krifka does, this isnot what MAX gives us, since e 3 , the sum of e 1 and e 2 , is due to the cumulativityin the extension of j sleep (for John sleep) too. But if j sleep holds of e 3and e 1 is a proper part of e 3 , then MAX(j sleep) does not hold of e 1 . Butthen MAX does not do what it should do. Note that the argument, and hencethe need for complication, rests on the assumption that unbounded predicatesare cumulative. Given that I don’t share this assumption, but instead defineboundedness in terms of (partial) divisivity (99), I can say that e 3 in thisscenario is not in the extension of j sleep (something which is not against ourintuitions). For this reason I can stick with the simple maximality operatorMAX, which is a welcome result, as the notion of convexity for eventualities thatis involved in the complex definition is conceptually unclear (recall that Krifkahimself doesn’t provide a definition). I will return to MAX in the next section.Let’s first consider the effect of the maximality operator in combinationwith the semantics of the aorist. The intervention of the maximality coercion