Aspect in Ancient Greek - Nijmegen Centre for Semantics

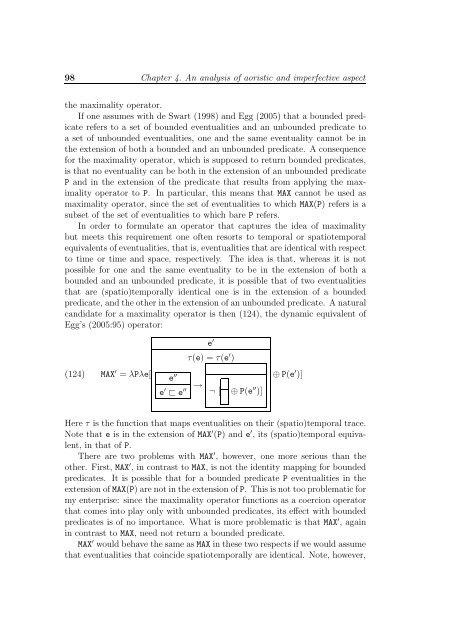
98 Chapter 4. An analysis of aoristic and imperfective aspectthe maximality operator.If one assumes with de Swart (1998) and Egg (2005) that a bounded predicaterefers to a set of bounded eventualities and an unbounded predicate toa set of unbounded eventualities, one and the same eventuality cannot be inthe extension of both a bounded and an unbounded predicate. A consequencefor the maximality operator, which is supposed to return bounded predicates,is that no eventuality can be both in the extension of an unbounded predicateP and in the extension of the predicate that results from applying the maximalityoperator to P. In particular, this means that MAX cannot be used asmaximality operator, since the set of eventualities to which MAX(P) refers is asubset of the set of eventualities to which bare P refers.In order to formulate an operator that captures the idea of maximalitybut meets this requirement one often resorts to temporal or spatiotemporalequivalents of eventualities, that is, eventualities that are identical with respectto time or time and space, respectively. The idea is that, whereas it is notpossible for one and the same eventuality to be in the extension of both abounded and an unbounded predicate, it is possible that of two eventualitiesthat are (spatio)temporally identical one is in the extension of a boundedpredicate, and the other in the extension of an unbounded predicate. A naturalcandidate for a maximality operator is then (124), the dynamic equivalent ofEgg’s (2005:95) operator:e ′τ(e) = τ(e ′ )(124) MAX ′ = λPλe[e ′′e ′ ⊏ e ′′ → ¬ [ ⊕P(e ′′ )]⊕P(e ′ )]Here τ is the function that maps eventualities on their (spatio)temporal trace.Note that e is in the extension of MAX ′ (P) and e ′ , its (spatio)temporal equivalent,in that of P.There are two problems with MAX ′ , however, one more serious than theother. First, MAX ′ , in contrast to MAX, is not the identity mapping for boundedpredicates. It is possible that for a bounded predicate P eventualities in theextension ofMAX(P) are not in the extension ofP. This is not too problematic formy enterprise: since the maximality operator functions as a coercion operatorthat comes into play only with unbounded predicates, its effect with boundedpredicates is of no importance. What is more problematic is that MAX ′ , againin contrast to MAX, need not return a bounded predicate.MAX ′ would behave the same asMAX in these two respects if we would assumethat eventualities that coincide spatiotemporally are identical. Note, however,