Proceedings of the 44th Symposium on Ring Theory and ...
Proceedings of the 44th Symposium on Ring Theory and ...
Proceedings of the 44th Symposium on Ring Theory and ...
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
Remark 6. For i = 1, . . . , 4, let Λ i = KΓ i /I i be <str<strong>on</strong>g>the</str<strong>on</strong>g> cluster-tilted algebra <str<strong>on</strong>g>of</str<strong>on</strong>g> type D n<br />
corresp<strong>on</strong>ding to Γ i . Then we see from [3, 24] that<br />
(1) Λ 1 is <str<strong>on</strong>g>the</str<strong>on</strong>g> path algebra <str<strong>on</strong>g>of</str<strong>on</strong>g> a Dynkin quiver <str<strong>on</strong>g>of</str<strong>on</strong>g> type D m .<br />
(2) Λ 2 is <str<strong>on</strong>g>of</str<strong>on</strong>g> type D 4 , <strong>and</strong> I 2 = 〈a 1 a 2 , b 1 b 2 , a 2 a 0 , b 2 b 0 , a 0 a 1 − b 0 b 1 〉. We immediately see<br />
that Λ 2 is a special biserial algebra <str<strong>on</strong>g>of</str<strong>on</strong>g> [22], but not a self-injective algebra.<br />
(3) Λ 3 is <str<strong>on</strong>g>of</str<strong>on</strong>g> type D m , <strong>and</strong> I 3 = 〈p | p is a path <str<strong>on</strong>g>of</str<strong>on</strong>g> length m − 1〉. Hence Λ 3 is a<br />
(m−1, 1)-stacked m<strong>on</strong>omial algebra, <strong>and</strong> is also a self-injective Nakayama algebra.<br />
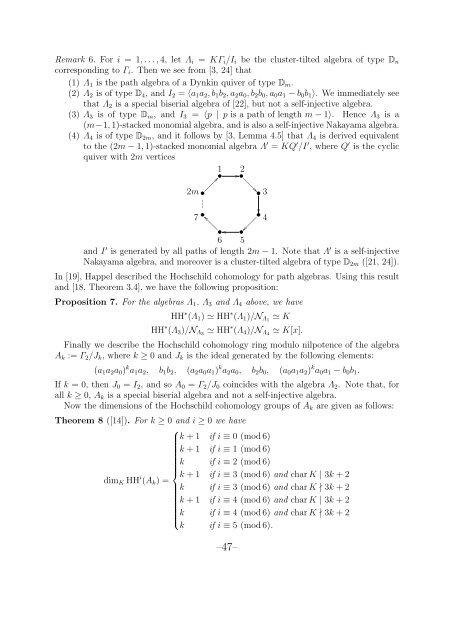
(4) Λ 4 is <str<strong>on</strong>g>of</str<strong>on</strong>g> type D 2m , <strong>and</strong> it follows by [3, Lemma 4.5] that Λ 4 is derived equivalent<br />
to <str<strong>on</strong>g>the</str<strong>on</strong>g> (2m − 1, 1)-stacked m<strong>on</strong>omial algebra Λ ′ = KQ ′ /I ′ , where Q ′ is <str<strong>on</strong>g>the</str<strong>on</strong>g> cyclic<br />
quiver with 2m vertices<br />
1 2<br />
• • ❄ ❄❄❄❄<br />
2m 8<br />
•<br />
8888 • 3<br />
.<br />
7 • ❄ ❄❄❄❄ • 4<br />
• 8<br />
•<br />
8888<br />
6 5<br />
<strong>and</strong> I ′ is generated by all paths <str<strong>on</strong>g>of</str<strong>on</strong>g> length 2m − 1. Note that Λ ′ is a self-injective<br />
Nakayama algebra, <strong>and</strong> moreover is a cluster-tilted algebra <str<strong>on</strong>g>of</str<strong>on</strong>g> type D 2m ([21, 24]).<br />
In [19], Happel described <str<strong>on</strong>g>the</str<strong>on</strong>g> Hochschild cohomology for path algebras. Using this result<br />
<strong>and</strong> [18, Theorem 3.4], we have <str<strong>on</strong>g>the</str<strong>on</strong>g> following propositi<strong>on</strong>:<br />
Propositi<strong>on</strong> 7. For <str<strong>on</strong>g>the</str<strong>on</strong>g> algebras Λ 1 , Λ 3 <strong>and</strong> Λ 4 above, we have<br />
HH ∗ (Λ 1 ) ≃ HH ∗ (Λ 1 )/N Λ1 ≃ K<br />
HH ∗ (Λ 3 )/N Λ3 ≃ HH ∗ (Λ 4 )/N Λ4 ≃ K[x].<br />
Finally we describe <str<strong>on</strong>g>the</str<strong>on</strong>g> Hochschild cohomology ring modulo nilpotence <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> algebra<br />
A k := Γ 2 /J k , where k ≥ 0 <strong>and</strong> J k is <str<strong>on</strong>g>the</str<strong>on</strong>g> ideal generated by <str<strong>on</strong>g>the</str<strong>on</strong>g> following elements:<br />
(a 1 a 2 a 0 ) k a 1 a 2 , b 1 b 2 , (a 2 a 0 a 1 ) k a 2 a 0 , b 2 b 0 , (a 0 a 1 a 2 ) k a 0 a 1 − b 0 b 1 .<br />
If k = 0, <str<strong>on</strong>g>the</str<strong>on</strong>g>n J 0 = I 2 , <strong>and</strong> so A 0 = Γ 2 /J 0 coincides with <str<strong>on</strong>g>the</str<strong>on</strong>g> algebra Λ 2 . Note that, for<br />
all k ≥ 0, A k is a special biserial algebra <strong>and</strong> not a self-injective algebra.<br />
Now <str<strong>on</strong>g>the</str<strong>on</strong>g> dimensi<strong>on</strong>s <str<strong>on</strong>g>of</str<strong>on</strong>g> <str<strong>on</strong>g>the</str<strong>on</strong>g> Hochschild cohomology groups <str<strong>on</strong>g>of</str<strong>on</strong>g> A k are given as follows:<br />
Theorem 8 ([14]). For k ≥ 0 <strong>and</strong> i ≥ 0 we have<br />
⎧<br />
k + 1 if i ≡ 0 (mod 6)<br />
k + 1 if i ≡ 1 (mod 6)<br />
k if i ≡ 2 (mod 6)<br />
⎪⎨<br />
dim K HH i k + 1 if i ≡ 3 (mod 6) <strong>and</strong> char K | 3k + 2<br />
(A k ) =<br />
k if i ≡ 3 (mod 6) <strong>and</strong> char K ∤ 3k + 2<br />
k + 1 if i ≡ 4 (mod 6) <strong>and</strong> char K | 3k + 2<br />
k if i ≡ 4 (mod 6) <strong>and</strong> char K ∤ 3k + 2<br />
⎪⎩<br />
k if i ≡ 5 (mod 6).<br />
–47–