statistique, théorie et gestion de portefeuille - Docs at ISFA

12.2. Minimiser l’impact des grands co-mouvements 383
Cutting edge l
Portfolio tail risk
1. Tail dependence versus correlation
λ
λ
1.0
0.9
0.8
0.7
0.6
0.5
0.4
0.3
0.2
0.1
0
–1.0 –0.8 –0.6 –0.4 –0.2 0
ρ
0.2 0.4 0.6 0.8 1.0
1.0
0.9
0.8
0.7
0.6
0.5
0.4
0.3
0.2
0.1
Tail index ν = 3
Tail index ν = 10
0
–1.0 –0.8 –0.6 –0.4 –0.2 0
ρ
0.2 0.4 0.6 0.8 1.0
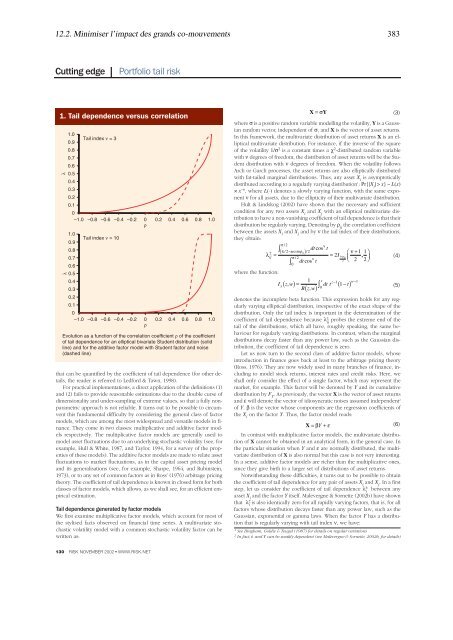
Evolution as a function of the correlation coefficient ρ of the coefficient
of tail dependence for an elliptical bivariate Student distribution (solid
line) and for the additive factor model with Student factor and noise
(dashed line)
that can be quantified by the coefficient of tail dependence (for other details,
the reader is referred to Ledford & Tawn, 1998).
For practical implementations, a direct application of the definitions (1)
and (2) fails to provide reasonable estimations due to the double curse of
dimensionality and under-sampling of extreme values, so that a fully nonparametric
approach is not reliable. It turns out to be possible to circumvent
this fundamental difficulty by considering the general class of factor
models, which are among the most widespread and versatile models in finance.
They come in two classes: multiplicative and additive factor models
respectively. The multiplicative factor models are generally used to
model asset fluctuations due to an underlying stochastic volatility (see, for
example, Hull & White, 1987, and Taylor, 1994, for a survey of the properties
of these models). The additive factor models are made to relate asset
fluctuations to market fluctuations, as in the capital asset pricing model
and its generalisations (see, for example, Sharpe, 1964, and Rubinstein,
1973), or to any set of common factors as in Ross’ (1976) arbitrage pricing
theory. The coefficient of tail dependence is known in closed form for both
classes of factor models, which allows, as we shall see, for an efficient empirical
estimation.
Tail dependence generated by factor models
We first examine multiplicative factor models, which account for most of
the stylised facts observed on financial time series. A multivariate stochastic
volatility model with a common stochastic volatility factor can be
written as:
130 RISK NOVEMBER 2002 ● WWW.RISK.NET
where σ is a positive random variable modelling the volatility, Y is a Gaussian
random vector, independent of σ, and X is the vector of asset returns.
In this framework, the multivariate distribution of asset returns X is an elliptical
multivariate distribution. For instance, if the inverse of the square
of the volatility 1/σ2 is a constant times a χ2-distributed random variable
with ν degrees of freedom, the distribution of asset returns will be the Student
distribution with ν degrees of freedom. When the volatility follows
Arch or Garch processes, the asset returns are also elliptically distributed
with fat-tailed marginal distributions. Thus, any asset Xi is asymptotically
distributed according to a regularly varying distribution1 : Pr{|Xi | > x} ~ L(x)
× x –ν , where L(⋅) denotes a slowly varying function, with the same exponent
ν for all assets, due to the ellipticity of their multivariate distribution.
Hult & Lindskog (2002) have shown that the necessary and sufficient
condition for any two assets Xi and Xj with an elliptical multivariate distribution
to have a non-vanishing coefficient of tail dependence is that their
distribution be regularly varying. Denoting by ρij the correlation coefficient
between the assets Xi and Xj and by ν the tail index of their distributions,
they obtain:
π / 2
ν
∫
dt cos t
± ( π/ 2−arcsin ρij
) / 2
ν + 1
λij
= = 2I
1+
ρ ,
π / 2
ij
(4)
ν
dt cos t
2 2
1 ⎛ ⎞
⎝
⎜
2⎠
⎟
where the function:
∫
0
X =σY
1
Ix( z, w)=
Bzw ( , )
( )
x z−1
w−1
dt t 1 − t
0
denotes the incomplete beta function. This expression holds for any regularly
varying elliptical distribution, irrespective of the exact shape of the
distribution. Only the tail index is important in the determination of the
coefficient of tail dependence because λ ± ij probes the extreme end of the
tail of the distributions, which all have, roughly speaking, the same behaviour
for regularly varying distributions. In contrast, when the marginal
distributions decay faster than any power law, such as the Gaussian distribution,
the coefficient of tail dependence is zero.
Let us now turn to the second class of additive factor models, whose
introduction in finance goes back at least to the arbitrage pricing theory
(Ross, 1976). They are now widely used in many branches of finance, including
to model stock returns, interest rates and credit risks. Here, we
shall only consider the effect of a single factor, which may represent the
market, for example. This factor will be denoted by Y and its cumulative
distribution by FY . As previously, the vector X is the vector of asset returns
and ε will denote the vector of idiosyncratic noises assumed independent2 of Y. β is the vector whose components are the regression coefficients of
the Xi on the factor Y. Thus, the factor model reads:
(6)
In contrast with multiplicative factor models, the multivariate distribution
of X cannot be obtained in an analytical form, in the general case. In
the particular situation when Y and ε are normally distributed, the multivariate
distribution of X is also normal but this case is not very interesting.
In a sense, additive factor models are richer than the multiplicative ones,
since they give birth to a larger set of distributions of asset returns.
Notwithstanding these difficulties, it turns out to be possible to obtain
the coefficient of tail dependence for any pair of assets Xi and Xj . In a first
step, let us consider the coefficient of tail dependence λ ± i between any
asset Xi and the factor Y itself. Malevergne & Sornette (2002b) have shown
that λ ± X = βY+ ε
i is also identically zero for all rapidly varying factors, that is, for all
factors whose distribution decays faster than any power law, such as the
Gaussian, exponential or gamma laws. When the factor Y has a distribution
that is regularly varying with tail index ν, we have:
1 See Bingham, Goldie & Teugel (1987) for details on regular variations
2 In fact, ε and Y can be weakly dependent (see Malevergne & Sornette, 2002b, for details)
∫
(3)
(5)