Numerical Methods Contents - SAM

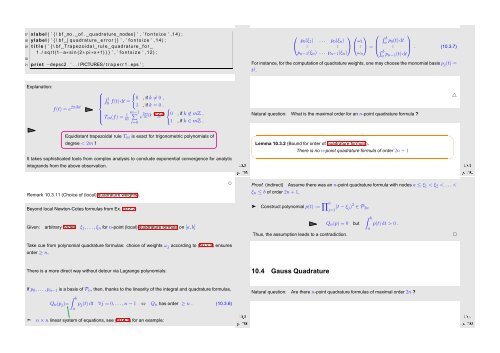
47 xlabel ( ’ { \ b f no . o f . quadrature nodes } ’ , ’ f o n t s i z e ’ ,14) ;
48 ylabel ( ’ { \ b f | quadrature e r r o r | } ’ , ’ f o n t s i z e ’ ,14) ;
49 t i t l e ( ’ { \ b f Trapezoidal r u l e quadrature f o r
1 . / s q r t (1−a∗ s i n (2∗ p i ∗x +1) ) } ’ , ’ f o n t s i z e ’ ,12) ;
50
51 p r i n t −depsc2 ’ . . / PICTURES/ t r a p e r r 1 . eps ’ ;
⎛
⎝ p ⎞ ⎛
0(ξ 1 ) ... p 0 (ξ n )
.
. ⎠ ⎝ ω ⎞ ⎛ ∫ ⎞ ba
1 p
. ⎠ ⎜ 0 (t) dt
⎟
= ⎝ .
∫
⎠ . (10.3.7)
p n−1 (ξ n ) ... p n−1 (ξ n ) ω n ba
p n−1 (t) dt
For instance, for the computation of quadrature weights, one may choose the monomial basis p j (t) =
t j .
Explanation:
{
∫ 1 0 , if k ≠ 0 ,
⎧⎪ ⎨ 0 f(t) dt =
f(t) = e 2πikt 1 , if k = 0 .
⎪ ⎩
T m (f) = m
1 m−1 ∑
l=0
e 2πi
m lk (7.2.2)
=
{
0 , if k ∉ mZ ,
1 , if k ∈ mZ .
Natural question: What is the maximal order for an n-point quadrature formula ?
△
Equidistant trapezoidal rule T m is exact for trigonometric polynomials of
degree < 2m !
It takes sophisticated tools from complex analysis to conclude exponential convergence for analytic
integrands from the above observation.
Ôº ½¼º¿
✬
Lemma 10.3.2 (Bound for order of quadrature formula).
There is no n-point quadrature formula of order 2n + 1
✫
✩
Ôº½ ½¼º¿
✪
Remark 10.3.11 (Choice of (local) quadrature weights).
Beyond local Newton-Cotes formulas from Ex. 10.2.2:
Given: arbitrary nodes ξ 1 ,...,ξ n for n-point (local) quadrature formula on [a,b]
✸
Proof. (indirect) Assume there was an n-point quadrature formula with nodes a ≤ ξ 1 < ξ 2 < ... <
ξ n ≤ b of order 2n + 1.
➤
Construct polynomial p(t) := ∏ n
j=1 (t − ξ j) 2 ∈ P 2n
Q n (p) = 0 but
Thus, the assumption leads to a contradiction.
∫ b
p(t) dt > 0 .
a
✷
Take cue from polynomial quadrature formulas: choice of weights ω j according to (10.2.2) ensures
order ≥ n.
There is a more direct way without detour via Lagrange polynomials:
10.4 Gauss Quadrature
If p 0 ,...,p n−1 is a basis of P n , then, thanks to the linearity of the integral and quadrature formulas,
∫ b
Q n (p j )= p j (t) dt ∀j = 0,...,n − 1 ⇔ Q n has order ≥ n . (10.3.6)
a
Ôº¼ ½¼º¿
➣ n × n linear system of equations, see (10.4.1) for an example:
Natural question: Are there n-point quadrature formulas of maximal order 2n ?
Ôº¾ ½¼º