pdf (it, 1477.913 KB, 1/26/10)

Esercizio 26.2. Dato il problema di Cauchy
⎧
⎪⎨
26. STUDI QUALITATIVI 135
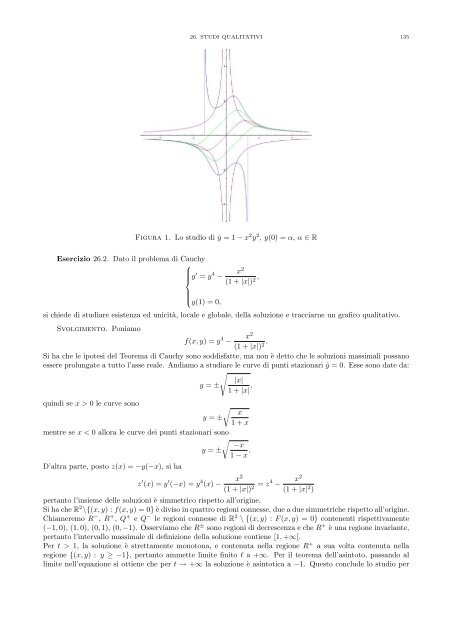
Figura 1. Lo studio di ˙y = 1 − x 2 y 2 , y(0) = α, α ∈ R
y ′ = y 4 −
⎪⎩
y(1) = 0,
x2 ,
(1 + |x|) 2
si chiede di studiare esistenza ed unicità, locale e globale, della soluzione e tracciarne un grafico qualitativo.
Svolgimento. Poniamo
f(x, y) = y 4 − .
(1 + |x|) 2
Si ha che le ipotesi del Teorema di Cauchy sono soddisfatte, ma non è detto che le soluzioni massimali possano
essere prolungate a tutto l’asse reale. Andiamo a studiare le curve di punti stazionari ˙y = 0. Esse sono date da:
y = ±
x 2
|x|
1 + |x| ,
quindi se x > 0 le curve sono
x
y = ±
1 + x
mentre se x < 0 allora le curve dei punti stazionari sono
−x
y = ±
1 − x .
D’altra parte, posto z(x) = −y(−x), si ha
z ′ (x) = y ′ (−x) = y 4 (x) −
x2 (1 + |x|) 2 = z4 x
−
2
(1 + |x| 2 )
pertanto l’insieme delle soluzioni è simmetrico rispetto all’origine.
Si ha che R 2 \{(x, y) : f(x, y) = 0} è diviso in quattro regioni connesse, due a due simmetriche rispetto all’origine.
Chiameremo R − , R + , Q + e Q − le regioni connesse di R 2 \ {(x, y) : F (x, y) = 0} contenenti rispettivamente
(−1, 0), (1, 0), (0, 1), (0, −1). Osserviamo che R ± sono regioni di decrescenza e che R + è una regione invariante,
pertanto l’intervallo massimale di definizione della soluzione contiene [1, +∞[.
Per t > 1, la soluzione è strettamente monotona, e contenuta nella regione R + a sua volta contenuta nella
regione {(x, y) : y ≥ −1}, pertanto ammette limite finito ℓ a +∞. Per il teorema dell’asintoto, passando al
limite nell’equazione si ottiene che per t → +∞ la soluzione è asintotica a −1. Questo conclude lo studio per