pdf (it, 1477.913 KB, 1/26/10)

32. MISCELLANEA DI ESERCIZI SUPPLEMENTARI 179
35
30
25
20
15
10
5
2 1 1 2
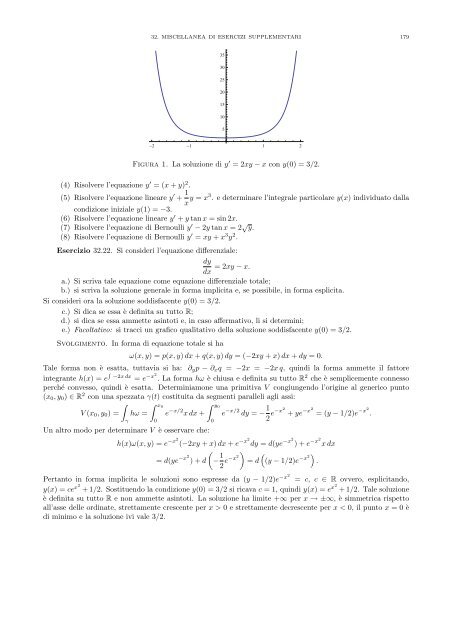
Figura 1. La soluzione di y ′ = 2xy − x con y(0) = 3/2.
(4) Risolvere l’equazione y ′ = (x + y) 2 .
(5) Risolvere l’equazione lineare y ′ + 1
x y = x3 . e determinare l’integrale particolare y(x) individuato dalla
condizione iniziale y(1) = −3.
(6) Risolvere l’equazione lineare y ′ + y tan x = sin 2x.
(7) Risolvere l’equazione di Bernoulli y ′ − 2y tan x = 2 √ y.
(8) Risolvere l’equazione di Bernoulli y ′ = xy + x 3 y 2 .
Esercizio 32.22. Si consideri l’equazione differenziale:
dy
= 2xy − x.
dx
a.) Si scriva tale equazione come equazione differenziale totale;
b.) si scriva la soluzione generale in forma implicita e, se possibile, in forma esplicita.
Si consideri ora la soluzione soddisfacente y(0) = 3/2.
c.) Si dica se essa è definita su tutto R;
d.) si dica se essa ammette asintoti e, in caso affermativo, li si determini;
e.) Facoltativo: si tracci un grafico qualitativo della soluzione soddisfacente y(0) = 3/2.
Svolgimento. In forma di equazione totale si ha
ω(x, y) = p(x, y) dx + q(x, y) dy = (−2xy + x) dx + dy = 0.
Tale forma non è esatta, tuttavia si ha: ∂yp − ∂xq = −2x = −2x q, quindi la forma ammette il fattore
integrante h(x) = e −2x dx −x = e 2
. La forma hω è chiusa e definita su tutto R2 che è semplicemente connesso
perché convesso, quindi è esatta. Determiniamone una primitiva V congiungendo l’origine al generico punto
(x0, y0) ∈ R2 con una spezzata γ(t) costituita da segmenti paralleli agli assi:
x0
V (x0, y0) = hω = e −x/2 y0
x dx +
γ
Un altro modo per determinare V è osservare che:
0
0
e −x/2 dy = − 1
2 e−x2
+ ye −x2
= (y − 1/2)e −x2
.
h(x)ω(x, y) = e −x2
(−2xy + x) dx + e −x2
dy = d(ye −x2
) + e −x2
x dx
= d(ye −x2
) + d − 1
2 e−x2
= d (y − 1/2)e −x2
.
Pertanto in forma implicita le soluzioni sono espresse da (y − 1/2)e−x2 = c, c ∈ R ovvero, esplicitando,
y(x) = cex2 + 1/2. Sostituendo la condizione y(0) = 3/2 si ricava c = 1, quindi y(x) = ex2 + 1/2. Tale soluzione
è definita su tutto R e non ammette asintoti. La soluzione ha limite +∞ per x → ±∞, è simmetrica rispetto
all’asse delle ordinate, strettamente crescente per x > 0 e strettamente decrescente per x < 0, il punto x = 0 è
di minimo e la soluzione ivi vale 3/2.